Location:
Hudson Beare, Classroom 4
Date:
Reza Jalali
Applications of Green-Naghdi equations in shallow water
Abstract:
Some oceanic phenomena cannot be solved without using a nonlinear, time domain model for fluid flow. For instance, the shoaling of a shallow water wave as it approaches a beach, and the transition of a group of steep deep water waves when they collide with an obstacle such as a vertical wall. The classical nonlinear equations of motion for the flow of an incompressible fluid are derived from mass and momentum-free balance leading to the continuity equation and Navier-Stokes momentum equation. Due to their nonlinearity, no general analytical solutions exist for the Navier-Stokes equation. Instead progress has been made using perturbation methods where by mathematical approximations one introduced to reduce the complexity of the equation system to the point where it can be solved. Green-Naghdi (GN) sheet theory, general Boussinesq (gB) equations and shallow water (SW) equations are in the middle of a spectrum between classic perturbation methods at one end and purely numerical technique at the other end. Like gB and SW equations, GN theory simplifies free surface flow problems by reducing them from three- to two-dimensions.
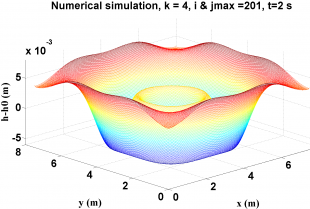
The present study is based on 1 and 2 D level I GN equations. It is worth mentioning that the present GN model is merely proposed for shallow water environment. Creating capable numerical solver of 2D level I GN equations is the exquisite part of thesis which has become possible after passing almost 40 years from the time that this theory was first developed by Green and Naghdi. For verification purposes, results present for sloshing of Gaussian hump in a rectangular basin. Furthermore, the simulations of 3D view of solitary wave propagation in an open channel, reverse simulations of Gaussian hump and simulations of even harmonics produced using 2D GN equations.
Mahdi Jalali
Curvilinear Model of Hydraulic Structures in Open Channels
Abstract:
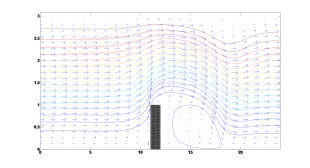
This PhD project aims at curvilinear system modelling of hydraulic structures (such as flood protection systems) in a circular channel containing wall-normal groins. The work is directly applicable to river engineering and river basin management. This study involves the following stages: development of a multi-grid solver of the curvilinear grid generation equations, derivation and numerical solution of the curvilinear shallow water equations, verification and validation of the solver against benchmark test cases (such as flow past a sidewall expansion, Fluid-Structure interaction and simulation of recirculation zone around groins), application to a demonstration case with associated parameter study for a test problem involving river flow past a curved border of Danube River, and analysis of uncertainty in the input parameters. This study is done from scratch using multi-grid solver of a form of curvilinear grid generation equation, which is demonstrated to be much more versatile than other iterative methods. The PhD research is of direct relevance to open channel flow hydraulics in environmental engineering.
Bio:
Reza and Mahdi are twin brothers with the same passions in the Engineering Sciences. They both received their Bsc degree in civil and hydraulics engineering. Because of their interest in solving mathematical problems in the Engineering Sciences they decided to continue their study in computational hydraulics. For his master’s degree, Reza simulated flownet of ground water based on 3D Laplace equations by applying Finite element method base of PDE tool box of Matlab. While for Mahdi’s masters, he studied the interface of water and soil in saturate and unsaturated Porous Media using Finite element method of Plaxis software. They both started their PhD in Jan 2013 at UCC (Ireland) under supervision of Professor Alistair Borthwick and they joined IES on Jan 2014. They are both members of the London and Edinburgh mathematic society and graduate members of the Institution of Civil Engineers.